Option pricing does, in fact, involve multiple aspects, each of which has a substantial impact on the cost of an option contract. The value of an option is determined not only by the current market price of the underlying asset, but also by a number of additional considerations. Here are the primary characteristics that influence option pricing, which are frequently incorporated into pricing models such as the Black-Scholes model for European options and the Binomial model for American options:
Underlying Asset’s Price
The price of the underlying asset has the greatest direct impact on the price of an option. Options are derivatives, which means their value is determined by the price of something else, in this case the underlying asset.
Call options often grow in value when the underlying asset’s price rises. This is because the call option gives the holder the right to buy the asset at a preset price (strike price), which increases in value when the market price rises.
For put options, the reverse is true. As the price of the underlying falls, the value of the put option rises since it gives the holder the opportunity to sell the asset at the strike price, which becomes more valuable as the market price drops.
Strike Price
The striking price is the price at which the option holder can buy (call) or sell (put) the underlying asset. The link between the underlying asset’s current price and the strike price influences an option’s intrinsic value. In the money (ITM) options are more expensive than at the money (ATM) or out of the money (ITM) options.
Time to Expiration
Although the concept of time’s effect is simple, recognizing its effects requires experience because of their expiration date. Good companies usually rise over lengthy periods of time, thus time is on the stock trader’s side. However, time works against the option buyer because the option’s value will decrease if days go by without a notable change in the underlying price.
Furthermore, an option’s value will decrease faster as its expiration date draws near. On the other hand, this is advantageous for the option seller, who aims to profit from time decay, particularly in the last month when it happens fastest.
Volatility
The amount of fluctuation in the underlying asset’s price over a certain period of time is measured by volatility. A high level of volatility indicates that an asset’s price is subject to abrupt swings, either upward or downward.
Options on extremely volatile assets carry a greater premium because of the increased risk and potential return. Vega in the Greeks is frequently used to symbolize volatility, which is a crucial element in options pricing models.
There are two different kinds of volatility: historical volatility, which is determined by previous price movements of the underlying asset, and implied volatility, which is represented by the market’s anticipation of future volatility and is reflected in the option’s current price.
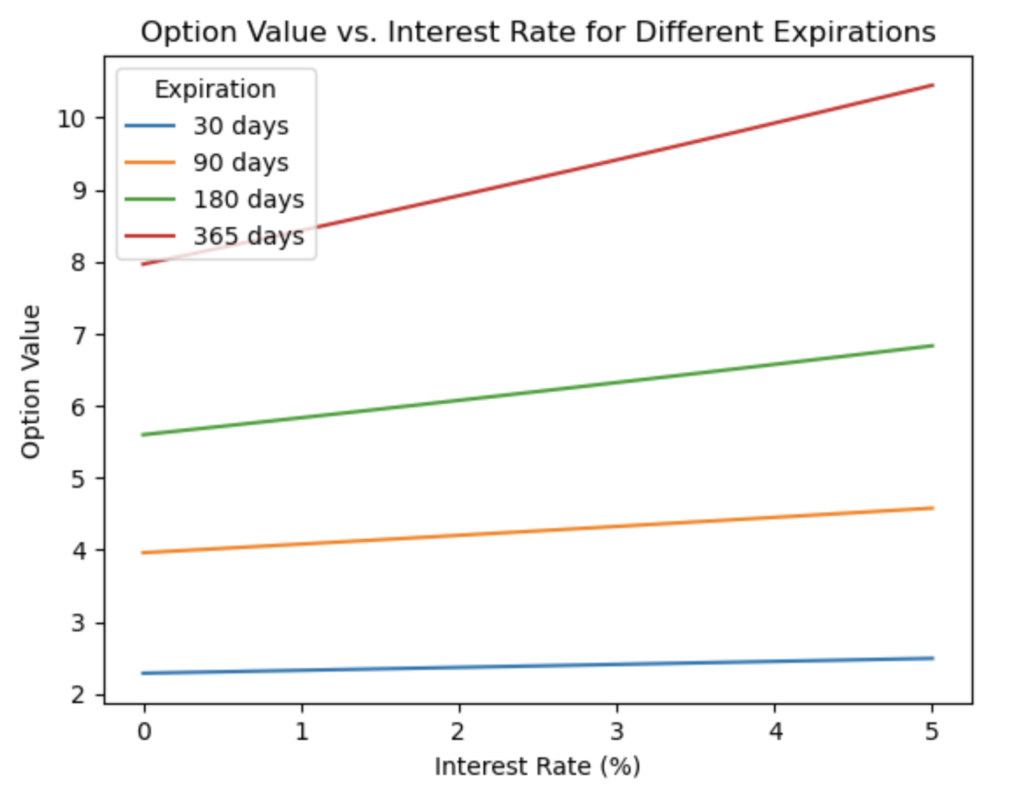
Interest Rates
Although it usually has a less noticeable impact than the other elements, the risk-free interest rate is another variable that might affect option prices.
The value of call options typically rises in response to an increase in interest rates since carrying positions in the underlying asset becomes more expensive. On the other hand, put options may lose value. This is due to the fact that the interest rate has an impact on the strike price’s present value that is paid (or received) at expiration.
The Rho in the Greeks, which gauges how sensitive the price of an option is to changes in interest rates, is frequently used to illustrate the impact of interest rates.
Dividends
Dividends from the underlying asset have an impact on option pricing even if they aren’t usually mentioned among the main variables. Anticipated payouts have the potential to drive down calls and raise put option prices, respectively.
This is due to the fact that a company’s stock price is anticipated to drop by the dividend amount when it pays out, which has an impact on call option payouts and increases the value of put options.
It is necessary for the trader to enter future volatility during the option’s life according to option pricing models. Naturally, option traders must make educated guesses by applying the pricing model “backwards” as they have no idea what it will be. After all, the trader already knows the price at which the option is trading, and with a little investigation, they can look at additional factors like interest rates, dividends, and remaining time. Consequently, future volatility, which may be calculated from other inputs, will be the sole value that is absent.